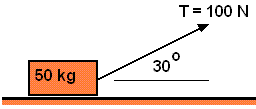
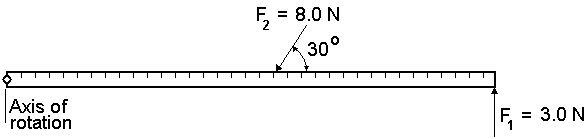One end of a meter stick is pinned to a table, so the stick
can rotate freely in a plane parallel to the tabletop. Two forces, both
parallel to the tabletop, are applied to the stick in such a way that the
net torque is zero. One force has a magnitude of 3.0 N and is applied
perpendicular to the length of the stick at the free end. The
other force has a magnitude of 8.00 N and acts at a 30.0o angle with
respect to the length of the stick as shown below.
Where along the stick is the 8.00 N force applied?
Express this distance with respect to the end that is pinned.