Simpson's Rule
If the function is represented by a quadratic function over the interval, the error is reduced further, Three points are required to form a quadratic function.
Figure 4 shows a function represented by a quadtatic over the interval. Since the quadratic has curvature a closer approximation is achieved and the error
is less.
When a function is represented by a quadratic the the approximate integral is,

The right hand side of the equation is the area under the approximate quadratic function.
When the interval is divided into n intervals and the integral is approximated by the sum of the intgrals over each small interval the
like the other methods, the accuracy increases. The magnitude of the error is less than,
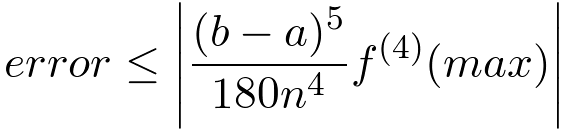
where f (4) is the maximum value of the forth derivative over the interval and n is the number of divisions of the interval (a ,b).
If the maximum values of the derivatives are known, the number of points, n, required to achieve an acceptable error can be calculated.
If the derivatives are not known,
it is usually possible to reduce the error to an acceptable value by using the trapazoidal method and increasing the number of points until the change in the
computed value of the integral is less than the required error limit.
Wikipedia has information on numerical integration.
